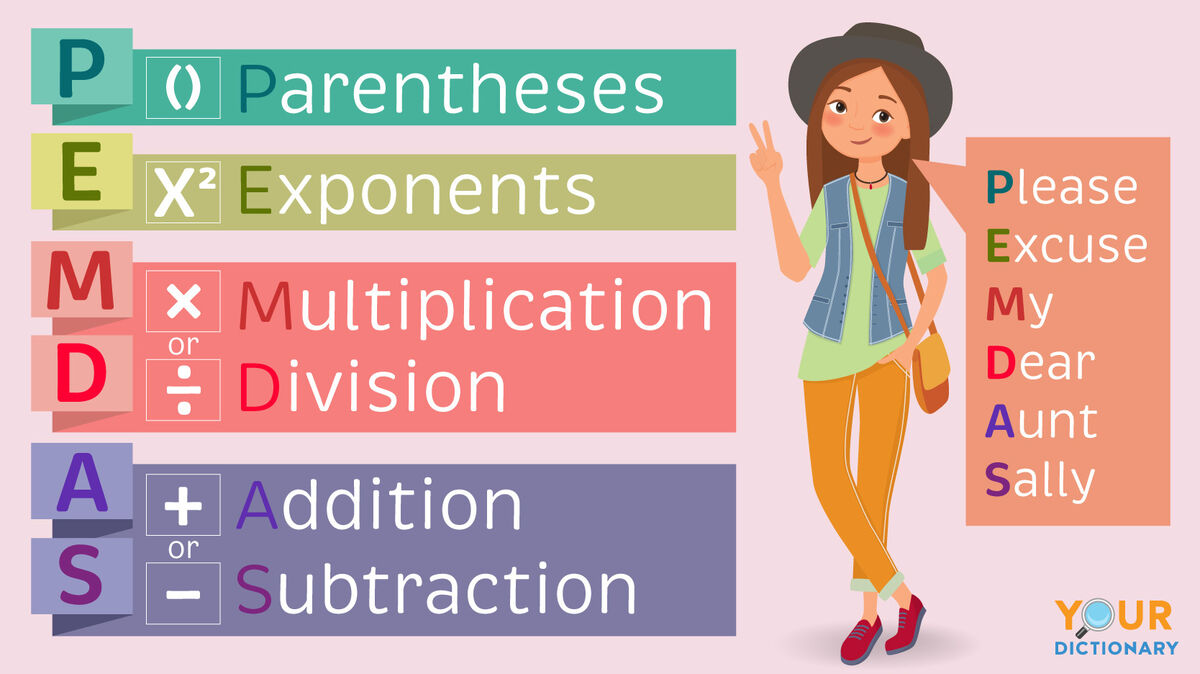
What does the phrase "Please excuse my dear Aunt Sally" have to do with math? It's a mnemonic that helps students remember PEMDAS — an acronym that outlines the order of operations rules in a math problem. Keep reading to learn the meaning of PEMDAS, what it stands for and why it's important when solving problems correctly.
PEMDAS Meaning
In English, you're used to reading from left to right. You solve math problems in a similar way — except for a few important steps. That's where PEMDAS comes in. But what does PEMDAS stand for? Each letter in the acronym stands for a mathematical operation:
- Parentheses
- Exponents and square roots
- Multiplication or Division
- Addition or Subtraction
Note that even though M (multiplication) comes before D (division), you don't necessarily complete multiplication before division. The same rule applies to addition and subtraction. You solve whichever operation comes first when reading from left to right.
Solving Problems With PEMDAS
The steps in PEMDAS indicate the order in which you solve a math problem.
For example, in the equation 6 x (9-2) + 52, you can separate the parts like this:
6 x | (9-2) | + | 52 |
| multiplication | parentheses | addition | exponents |
If you solve from left to right and ignore the order of operations, the answer to this equation would be 3249 (6 x 9 = 54, 54-2 = 52, 52 + 5 = 57, 572 = 3249), which is incorrect.
PEMDAS directs you to work in this order:
- Solve within all parentheses (P): (9-2) = 7
- Solve all exponents/powers (E): 52 = 25
- Solve all multiplication OR division (M or D), left to right: 6 x 7 = 42
- Solve all addition OR subtraction (A or S), left to right: 42 + 25 = 67
Using PEMDAS, we can determine that 6 x (9-2) + 52 = 67, which is the correct answer. You can see what a big difference there is when you use the proper order of operations!
Example Problems Using PEMDAS
Does PEMDAS really work in every situation? Take a look at several more equations in which PEMDAS is necessary to find the correct answer.
This equation involves all the orders of operations in PEMDAS, including two sets of exponents. Just like in the other steps of PEMDAS, you solve these exponents left to right. Use the steps as follows:
- Solve parentheses: 83 ÷ (2) - 42
- Solve exponents: 512 ÷ 2 - 16
- Solve multiplication OR division, left to right: 256 - 16
- Solve addition OR subtraction, left to right: 240
When you see a number in front of a parenthesis, you multiply the solution of the parentheses by that number. In this equation, you'd multiply (7 x 4) by 3, and (2 x 5) by 8. But the rules of PEMDAS indicate that you need to solve the parentheses (and square the solution of the first parentheses) before you multiply. It goes like this:
- Solve parentheses: 3(28)2 + 8(10)
- Solve exponents: 3(784) + 8(10)
- Solve multiplication OR division, left to right: 2352 + 80
- Solve addition OR subtraction, left to right: 2432
Some students panic when they see parentheses inside parentheses. What does PEMDAS say about that? Work from the inside out — that is, solve the innermost set of parenthesis, then work out to the outer parentheses.
- Solve inner parentheses: 100 ÷ 52 + (20 - (15))
- Solve outer parentheses: 100 ÷ 52 + (5)
- Solve exponents: 100 ÷ 25 + (5)
- Solve multiplication OR division, left to right: 4 + (5)
- Solve addition OR subtraction, left to right: 9
What do you do when there's a square root in the mix? Square roots fall into the same step as exponents. You solve them after solving parentheses and before dividing or multiplying.
- Solve parentheses: 152 + √9 - (12)2 ÷ 2
- Solve exponents and square root, left to right: 225 + 3 - 144 ÷ 2
- Solve multiplication OR division, left to right: 225 + 3 - 72
- Solve addition OR subtraction, left to right: 156
An exponent inside parentheses can lead to much confusion. But remember, the rules of PEMDAS still apply inside the parentheses, so the exponents come before the multiplication.
- Solve exponents inside parentheses: 103 + 2(625) ÷ 5(1 x 1)
- Solve parentheses: 103 + 2(625) ÷ 5(1)
- Solve exponents outside parentheses: 1000 + 2(625) ÷ 5(1)
- Solve multiplication OR division, left to right: 1000 + 250
- Solve addition OR subtraction, left to right: 1250
Did you get the right answers? Remember that all operations inside of parentheses come before every other operation. If there are no parentheses or exponents, you simply skip those steps and multiply or divide from left to right, then add or subtract from left to right.
Other Acronyms for Order of Operations
If the concept of the order of operations is familiar to you, but PEMDAS isn't, you might have grown up with a different acronym. Other acronyms to explain the order of operations include:
- BEDMAS (Brackets, Exponents, Division, Multiplication, Addition, Subtraction)
- BODMAS (Brackets, Order, Division, Multiplication, Addition, Subtraction)
- GEMDAS (Grouping, Exponents, Multiplication, Division, Addition, Subtraction)
All of these acronyms explain the same process with different words. GEMDAS seems like it's different from the others, but "grouping" is another word for "parentheses" or "brackets." It also switches Multiplication and Division, but as we know, you use these operations in the same step anyway.
More Math Acronyms and Abbreviations
Acronyms such as PEMDAS help young learners (and experienced mathematicians) remember the basic steps of solving equations with more than one operation. Learn more about mnemonics and how they help us learn with these helpful examples of mnemonics. You can also master common measurement abbreviations for common units before starting on important geometry concepts.